
Damn, it sure takes a long time for light to travel to the Pegasus galaxy. If only quantum teleportation enabled FTL Stargates…
I was hoping to post this earlier, but a heavy dose of writer’s block set in (I met a girl, and no, this blog didn’t help — but she is a physicist!) I also got lost in the rabbit hole that is quantum teleportation. My initial intention with this series of posts was simply to clarify common misconceptions and to introduce basic concepts in quantum information. However, while doing so, I started a whirlwind tour of deep questions in physics which become unavoidable as you think harder and deeper about quantum teleportation. I’ve only just begun this journey, but using quantum teleportation as a springboard has already led me to contemplate crazy things such as time-travel via coupling postselection with quantum teleportation and the subtleties of entanglement. In other words, quantum teleportation may not be the instantaneous Stargate style teleportation you had in mind, but it’s incredibly powerful in its own right. Personally, I think we’ve barely begun to understand the full extent of its ramifications.
Beyond experimentally verifying that zero probability events can indeed occur (myself meeting a girl), the other reason this post took so long, is because aspects of the teleportation protocol are extremely subtle. I got bogged down in my first draft of this post by trying to carefully explain the trickiest aspects of teleportation. In particular, trying to answer whether or not the protocol truly has instantaneous/nonlocal/superluminal aspects. The short answer is probably — but that we’re not positive. In order to answer this question more concretely, I would need to introduce the concepts of locality and realism, and then to explain the highlights of Einstein, Podolsky and Rosen’s 1935 paper which advocated for local realism, and then Bell’s 1964 paper which showed that no theory can preserve both locality and realism while simultaneously matching the experimental results of quantum mechanics. I might cover these subtleties in a future post, but for now, I’m going to bring you straight to the cutting edge of teleportation experiments and then I’ll explain the teleportation protocol in more detail.
My last two posts introduced quantum bits (qubits) and entanglement. Qubits (superpositions of 0s and 1s) are the fundamental units in quantum information — their role is similar to that of bits (0s and 1s) in classical information theory. As a brief review of notation, we write qubits in the bra-ket notation, where an arbitrary qubit can be written as . The fact that the fundamental units in quantum information are superpositions of two states should hint at the power of quantum computation over classical computation — instead of being restricted to 0s or 1s, the input to a quantum computer is a superposition of these two states, and can therefore explore multiple possible outcomes in parallel.
Entanglement is the idea that we can generate pairs of qubits which are correlated in powerful ways. For our purposes, we can consider the maximally entangled pair (maximally entangled means that if you know the state of one qubit, then you know the state of the other.) In more detail, this state corresponds to two qubits, where Alice and Bob each have one qubit from the pair. This next point is important: the fact that Alice and Bob’s qubits are maximally entangled means that once one qubit has been determined, then the value of the other is also known. This is true even if the measurements are in different bases (ex: measure atomic spin along x or z axes) — or if the measurements are space-like separated. One mechanism which allows physicists to generate entangled qubits in the real world (not just in theorists’ kooky brains) is the effect of spontaneous parametric down-conversion which says that inside a nonlinear crystal, an incoming photon can spontaneously decay into two maximally entangled photons (where the entanglement is in terms of the photon polarization.)
Quantum teleportation is a recent idea (on the timescale of breakthroughs in physics.) Also, please forgive me if I have any of the history wrong, but to the best of my knowledge, the protocol was discovered in 1993 by a team of six researchers (mainly at IBM), and then experimentally verified for the first time in 1997 by a team of physicists working in the UK and Italy. Steady improvements in techniques and equipment since then culminate in the recent experiments at Qinghai Lake in Western China and in the Canary Islands, which I’ll describe shortly. Before doing so, I want to emphasize why you should care about quantum teleportation. Here are two things that quantum teleportation does not allow followed by three of its crowning achievements:
- NO-FTL-communication: quantum teleportation does NOT enable faster-than-light communication. To the best of our current knowledge, this is still regarded as impossible.
- NO-cloning: quantum teleportation does NOT enable us to clone unknown quantum states. There is a foundational result in quantum information, the no-cloning theorem, which says that it’s impossible to build a machine whose input is one unknown qubit and one known qubit, and then whose output is two copies of the unknown qubit.
- Transmission of unknown quantum information: quantum teleportation provides a mechanism to move unknown quantum states throughout the universe using two easier subtasks: distributing maximally entangled qubit pairs and classical communication (0s and 1s using light pulses.) It’s hard to describe how useful this is. Imagine being told about the internet in 1950 and trying to understand what it would be used for. It’s kind of like that (in my heavily biased opinion.) Any semblance of a quantum internet will intimately rely upon teleportation.
- Uninterrupted quantum internet service providers: in order for quantum teleportation to work, we need to distribute entangled qubits between Alice and Bob. We need a quantum channel between them in order to do so. However, this channel doesn’t always need to be operating properly — when it’s online, we can send extra entangled pairs and thereby build up a reservoir for future use. Also, it doesn’t need to be able to reliably transmit arbitrary qubits, it only needs to be able to transmit one type of maximally entangled pairs. Imagine how useful it would be if your current ISP were this reliable 🙂
- Security/broadcasting: quantum teleportation is integral in many quantum cryptography protocols. Moreover, one interesting aspect of the quantum teleportation protocol is that (as you’re about to see) Alice doesn’t need to know where Bob is located in order to teleport him an unknown qubit. All that is required is that she performs a measurement on her two qubits and then broadcasts a classical message detailing her measurement outcome. Only Bob, with his entangled qubit in hand, will be able to use the message to recover Alice’s original unknown qubit.
Where we’re at experimentally:
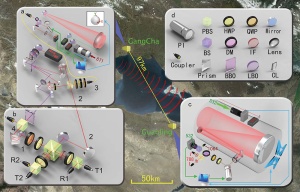
1. Two experiments were recently performed at Qinghai Lake in Western China (arXiv). The first experiment involves quantum teleportation in open air at a distance of 97km. The researchers set up two laboratories 97km apart. They generated an entangled photon pair at Alice’s laboratory. They then distributed one of the qubits from this entangled pair to Bob’s lab, 97km away. The core technologies that enabled this achievement aren’t particularly special in there own right, but rather in the way that they were used in tandem. Basically, the team used a smorgasbord of lasers operating at different wavelengths and tools from classical optics, such as beamsplitters and mirrors. The advantage of not using fiber optics, is that photon loss and decoherence are negligible in open air (in contrast to decoherence being large when bouncing photons through fiber optic cables). Basically, the group was able to operate at an extreme level of precision, and to thereby set a new distance record for quantum teleportation.
The second experiment they performed involved distributing entanglement. In the scheme above, the entangled pair was generated in Alice’s lab, so they only needed to transmit one photon from the pair, and that was to Bob. In this experiment, they also took on the more difficult task of generating the entangled pair at a third laboratory, and then transmitting one photon to each of Alice and Bob’s laboratories. Moreover, they used their setup to “close the locality loophole” and to therefore provide experimental verification that both locality and realism cannot hold simultaneously. In this experiment, it takes 300 microseconds for light to travel between Alice and Bob’s labs, but only 3 microseconds for Alice and Bob to perform a series of measurements, meaning they were able to measure their photons in sequence before light could travel from Alice to Bob, and then later (limited by the speed of light) confirm that they always achieved the same outcome (and that the correlations of their entangled photons obey Bell’s theorem when they measure in different bases.)
2. A distance record was recently achieved in the Canary Islands (arXiv) by teleporting qubits 143km between the islands of La Palma and Tenerife. The Canary Islands paper describes the primary difference between their approach and the one used at Qinghai Lake as: “the most significant distinction between ref. 33 (Qinghai Lake) and the experiment presented in this Letter is our implementation of an active feed-forward technique.” What they mean by active feed forward wasn’t perfectly clear to me, but I’m under the impression that the distinction comes from changing the order in which the teleportation protocol unfolds (described below.) Basically, it seems like the Canary Islands team performed Step 3 before Step 2, meaning that Alice measured her two qubits before distributing an entangled qubit to Bob. The benefit of this is that after measuring, she simultaneously sends the entangled qubit (quantum channel) and her measurement outcome (classical channel) to Bob. Bob then, in real time, as the qubit and bits arrive, chooses which quantum logic gate to send his qubit through in order to recover the original unknown state of Alice’s qubit. The Canary Islands approach was also the first to create a particular mash-up involving a few other techniques: “to achieve this, we combine advanced techniques involving a frequency-uncorrelated polarization-entangled photon pair source, ultra-low-noise single-photon detectors and entanglement-assisted clock synchronization.” In summary, they combined a bunch of different tricks and tools in order to reliably perform quantum teleportation 143km through open air.
One of the exciting things about these two papers is that they both robustly performed teleportation through open air at a distance comparable to what is needed to teleport quantum information to satellites in low Earth orbit. Both papers mentioned satellite based quantum communication, and I therefore wouldn’t be surprised if experiments in the next few years demonstrate quantum teleportation between Earth-based labs and LEO satellites. In other words, much progress has been made, especially considering that quantum teleportation was only discovered twenty years ago!
I won’t blame you if you stop here, but for those of you who can stomach more details, here’s the protocol broken into steps:
Step 0: Set the stage. Two physicists, Alice and Bob, set up laboratories far apart, from which they will perform quantum teleportation. Alice has an unknown qubit that she would like to teleport to Bob. The fact that this qubit is unknown is important — if she knew the state of the qubit, then there are other ways that she could transmit the relevant quantum information.
Step 1: Generate a maximally entangled pair. Alice and Bob choose their favorite experimental trick to generate a maximally entangled pair of qubits
Step 2: Distribute the entangled pair between Alice and Bob. After generating the entangled pair, Alice and Bob need to somehow distribute the two qubits so that they each have one. In the recent Qinghai lake experiment, the entangled pair was generated in Alice’s laboratory and then one qubit from the pair was transmitted to Bob, using a sequence of lasers. Now that Alice and Bob each have one qubit, we can write the entangled pair as where I added subscripts to indicate who has which qubit.
Step 3: Alice changes her measurement basis. Alice’s goal is to transmit the unknown qubit to Bob. In order to teleport
, we need to consider the joint state of our three qubit system. This involves a bit of notation, but your intuition for what these symbols mean should be correct. The three qubit state is described as:
, which expands to:
I apologize if you need to squint too much — it’s fantastic that WordPress enables LaTeX — but it’s only a very light version! Also, I wasn’t very judicious with my tensor product symbols (
), but basically, between every pair of qubits, there should be an
symbol.
The mechanism that will actually affect Bob’s qubit is for Alice to perform a measurement on her two qubits in the Bell basis. By doing so, she collapses the state of her qubits and affects Bob’s as well. Instead of performing this measurement in the computational basis (which corresponds to the form originally written above), she rearranges terms and measures in the Bell basis. The concept of measurement bases is extremely important in quantum information, but I don’t have time to go through all of the associated details, so I’m going to leave this fuzzy. Besides, John Preskill’s free lecture notes cover qubits and measurement bases better than I ever could. Thus, Alice changes to the Bell basis which is described by the four basis states:
We can rewrite the four basis states corresponding to computational basis measurements using the substitutions:
You can see that these are the correct change of basis formulas by plugging in the definitions of and
, and then simplifying with algebra. If we plug in these values, we see that our state
can be written as:
We can then collect like terms to obtain:
This equation may seem beastly, but really all it’s saying is that Alice and Bob are sharing three qubits amongst themselves, Alice rotated her measurement apparatus to align with the Bell basis, and by doing so, the entangled state of their qubits was transformed to what’s written above. If Alice were to perform a measurement of in the Bell basis, then she is equally likely (probability 1/4) to get each of
,
,
or
For example, if Alice measured her two qubits and obtained
, then Bob’s qubit would be in the state
, where Bob still intends to measure his qubit with respect to the computational basis.
Step 4: Alice performs a measurement. The algebra in the section above shows that when Alice measures her two qubits in the Bell basis, she is equally likely to get: ,
,
or
Alice’s measurement affects Bob’s qubit. Depending on the outcome of Alice’s measurement, Bob’s qubit is now in the state:
,
,
, or
, respectively. This looks very similar to the state of the qubit
that Alice originally wanted to transmit to Bob, but it’s not quite there yet.
Step 5: Alice transmits classical information to Bob. Bob’s qubit is now in one of four possible states: ,
,
, or
, but he doesn’t know which. Alice sends two classical bits to Bob, describing the outcome of her measurement. She either sends 00 (
), 01 (
), 10 (
), or 11 (
). Bob can use this information, describing which measurement outcome Alice obtained, to transform his qubit into the state
This will happen in the next step. It is this transfer of classical information, limited by the speed of light, which leads to confusion about the fact that quantum teleportation does not enable instantaneous communication (the no-signaling theorem.)
Step 6: Bob performs an operation. Bob can use the information that Alice sent him to transform his qubit into the state . This relies upon the use of quantum logic gates, which take arbitrary qubits as input, and then perform an operation which depends upon this input. These are similar to logic gates in classical computing, where the inputs are usually streams of electrons and the outputs are also streams of electrons, but which depend upon the input (examples include AND, OR, NOT and NAND gates.) The two gates we need here are the X and Z gates. The Z gate changes the sign of the coefficient on the second basis item. For example, if you fed
into a Z gate, you would obtain
The X gate swaps the order of the two basis elements. For example, if you fed
into an X gate, you would obtain
Therefore Bob can use the following protocol to correct his qubit, based upon the measurement outcome that Alice obtained:
If 00 (), then do nothing (apply identity operation)
If 11 (), then apply a Z gate, which inverts the phase of the
coefficient
If 01 (), then apply an X gate which swaps
and
If 10 (), then apply an X gate followed by a Z gate. This transforms
to
and then to
.
After following this procedure, Bob’s qubit will be in the state , no matter which measurement outcome Alice obtained — we have successfully teleported Alice’s qubit to Bob using only a maximally entangled pair and classical communication!

A classical (and classic) approximation to the quantum dessert alluded to in my original post: cookie-brownie superposition!
Step 7: Bob now has Alice’s qubit. Bob’s qubit is in the state: . It really is in this superposition. It hasn’t yet collapsed to either
or
. It’s identical to the qubit that Alice originally wanted to transmit. Pop open the champagne or eat some real life cookie-brownie superposition ice cream!


If the quantum teleportation is successfully invented is there a possibility of time travel to the past?
nice question
Correct me if I’m wrong, the amount of time it will take to an object to teleport to one place to another is unknown do to the fact of space and time. For example in order to get your object to relocate in another place it would get lost because the object will be traveling through air, space and time. It might get trapped in another dimension. In other words if I make my own teleportation devise here at home and place one in my room and another in my living room and I place an apple in my room in hopes that it will travel to the living room is highly impossible. It will be destroyed instantly. What if my apple went to someone’s teleporter and accidentally instead of the one in my living room. Now how do I link my teleporter in order to create a line for the apple to travel and get there in one piece? Now if this “works” then how will traveling through time be possible? Wouldn’t life as we know it end? The amount of time and energy we are making for the time machine is impossible. We would get lost, and if I traveled to a time where I exist wouldn’t I die as well do to the pressure and lack of air? Remember we are traveling through “space and time”.
It is not travelling. It would be a copy of you if technology advanced enough. Your particle state’s reprinted in a human 3d printer. Transmitted through a signal to a receiver and reprinted.
dram is the only one who can teleport from kino der toten across map idiot
No it would be like data travelling on a median like a photon etc. They have slowed light down already and encoded on it if I’m correct.
JFAFF just got FUCKED BY FEDS YO, were sending it to dirams after the T’s, everybody is screwed.
You can’t get lost if you do your quidbits would have been scanned and saved. You would not be perfect but a clone made with other atoms will be possible in future. 75-125 years.
anyone know how to pack a punch my heroin needles?
On what map?
No not unknown. Entangled. Instantanious. No TIME in transit. Walk in the portal and walk out to the other end. Like nothing in between.
Yo jfaff is so screwed, he cant get a run tonight so were just gonna chief down his beer pods until we get to dirams. Full send.
According to my logic, I don’t know whether it is correct or not, but when you buid a Teleportation machine. There must be a sender and receiver or they act vise a versa. So if sender send a apple the machine must have a path towards a receiver, so no chance of misslocation. But if we consider the theory of quibits, it is get stuck in another dimension. The porpose for which machine is build, is same not be mispalce. And according to theory it get missplace but the base of machine building is to create a path for 1 to 2. And if this machine is build the photon can travel in miliseconds faster than light so the time travel principal is also satisfied.
Diram cant find his beer pods, so were sending it to GUT, dont tell the feds yo.
Im selling my Juul with 3 pods hmu i got a charger
Good day! I know this is kinda off topic but I was wondering if you knew where I could get a captcha plugin for my comment form?
I’m using the same blog platform as yours and I’m having trouble finding one?
Thanks a lot!
Pingback: Jeff Kimble wins 2013 Herbert Walther Award | Quantum Frontiers
Awesome explanation !
My spouse and I stumbled over here from a different page and thought I might
check things out. I like what I see so now i’m following you. Look forward to checking out your web page for a second time.
Thanks for taking the time to write such a fascinating post! Do you have a surplus ) in the expression that follows “which expands to”? Best of luck with the girl!
Matt
i will help you in your time travel experiment i want go back my friend please help
Good read.
Teleportation of software in the future might cause some problems.
No carrier and no sender info?
Countermeasures must be taken.
Infinite Gödel primes in Mandelbrot en Von Neumann tech. could be the solution.
Encryption must be really unbreakable (100 %) before there can be talk of implementation in consumer electronics.
I mean planting entangled qubits should be a crime.
Howdy just wanted to give you a quick heads up. The text in your article
seem to be running off the screen in Internet explorer.
I’m not sure if this is a format issue or something to do with internet
browser compatibility but I figured I’d post to let you know.
The design and style look great though! Hope you get the issue fixed
soon. Cheers
I know how to build a transport for teleportation but we will have to talk in person I don’t need this technology falling into the wrong hands
i want to get involved too. can I?
Hey
Are you relly serious about this.
And from what age you started to work to built the Teleportal machine,
And want to know little about you
My friend
Pingback: Clocking in at a Cambridge conference | Quantum Frontiers
Pingback: Beam me up, Scotty! How to build a quantum teleportation device | The Life of Psi
My family members all the time say that I am wasting my time here
aat net, except I know I am getting familiarity all tthe time by reading thes goood
posts.
Can you tell us more about this? I’d love to find out some additional information.
Really that’s how you do that I want to teleport to on place to onther.
equation are understandable
+u0+-.365258 fiths equation on how to make pods is 1 bleach + 2 heroin = dirams truck
it tried to they equation it get so so fucked. JFaff was so fucked
they back to past or future?
Diram is back to the past, but jr4score is completely fucked, I dont know how were gonna get a run yo his beer pod fake just got fucked. Send it to the ming theres zero feds.
I ѡant to to thank you for this very good read!!
I certainly enjoyed eνery bit of it. I Һave you saved as a ffavorite to look at new things you post…
Pingback: #TeletransporteCuántico #QuantumTeleportation | hotgirlandmore
Teleportation already exist,we do it every time on internet by watching movie,listening youtube, we stream and upload so may bites,MB,download GB,so my point is if we can transfer(read teleport) so many data we only need to figure out how to change atoms in ROM and vine-versa, human body contain 10(28)A and how much is that in data storage MB,GB;TB; so if we scan each atom individualy and it automatically become computer data and if we that data send to another computer and somehow re-scan it via projector in 4D would it be human teleportaton.
Easy exaple HUMAN=COMPUTER DATA =HUMAN
DRAM=DENT IN TRUCK+DRAM
Hey love your article. Fascinating work. I am a man of science myself. If at all possible, I would love to be one of the human experiment on this project if there’s ever gonna be one or already been made.
ill do it
What’s Taking place i’m new to this, I stumbled upon this I have found It absolutely useful
and it has helped me out loads. I hope to give a contribution & assist other customers like its helped me.
Good job.
i want to be in this
count me in
Pingback: Performing Bell State Measurement in Teleportation | Physics Forums - The Fusion of Science and Community
“your intuition for what these symbols mean should be correct” – lol, i’m absolutely sure my gut feeling is correct
I’ve been studying this for a long time, you can teleport objects. That’s not the issue. The real issue is that the object will not return. Now if you had 2 teloports that’s different. One one one end of the room and one other the other. One other issue is that if the object goes in it rips it to shreads. If you where to jump into it. Death
Pingback: Rsiran’s Abilitys – thedarkability
Pingback: How To Build A Teleporter | Tiriya5
Pingback: How To Build A Quantum Time Machine | Asia Bank
What before thinking of transferring atoms in teleporatation, must create the same frame dimension of the place we have to teleport as of the original dimension..
Nice article . There are a couple of questions I have :-
1) There are only two qubits which are entangled, right. The qubit with Alice which originally was in an unknown state is not entangled with the system. You are creating a product state, which can be factorized. So there are two entangled qubits here and another qubit which is independent but we are creating a product state with the system. Am I right?
2) Going through the steps , I kind of lost the vision of what we are trying to achieve. Classically, to send the information about the unknown qubit of Alice, we would need to make a measurement which would collapse the wavefunction and hence, the infornation about the original state cannot be sent to Bob. This is what we need to avoid. We want to send information about the ORIGINAl unknown qubit of Alice to Bob, right?
Recurb the ‘Nutrilic’whereisuponwhich ionic omni delay sequence/s (back,front,non linear/ linear ionic inversion
Maybe💪👀🍖
One must remeber that pie is an outcome of instances not the ither way around.
Food for thought..
Haooy new year 2 all
👊😎🍖888
Pingback: Taming Wave Functions with Neural Networks | Quantum Frontiers
Will it be possible to create a teleportation device within an app such as WhatsApp
No, unless you’ve got a room the size of a football field containing a quantum computer hooked up to an electromagnetic quantum gate and 15,000 watts of electricity.
BRO THIS IS TOO COMPLICATED! MAKE ONE AND SELL ON AMAZON! I PAY!
Make it and send me it pls or send me blueprints so idont explode like my cusin
I’m a string theorist from MIT, and I can say that this is very interesting.
Pingback: Teleportation Portal - CaLoginGuide
Pingback: Teleportation Portal Creation | Superpower Wiki | Fandom